heroeshaa.blogg.se - Joukowski airfoil

JOUKOWSKI AIRFOIL FREE

In the derivation of the Kutta–Joukowski theorem the airfoil is usually mapped onto a circular cylinder. At a large distance from the airfoil, the rotating flow may be regarded as induced by a line vortex (with the rotating line perpendicular to the two-dimensional plane). It should not be confused with a vortex like a tornado encircling the airfoil. This rotating flow is induced by the effects of camber, angle of attack and the sharp trailing edge of the airfoil. The fluid flow in the presence of the airfoil can be considered to be the superposition of a translational flow and a rotating flow. However, the circulation here is not induced by rotation of the airfoil. Kutta–Joukowski theorem relates lift to circulation much like the Magnus effect relates side force (called Magnus force) to rotation. Kutta–Joukowski theorem is an inviscid theory, but it is a good approximation for real viscous flow in typical aerodynamic applications. It is named after Martin Kutta and Nikolai Zhukovsky (or Joukowski) who first developed its key ideas in the early 20th century. The circulation is defined as the line integral around a closed loop enclosing the airfoil of the component of the velocity of the fluid tangent to the loop. The theorem relates the lift generated by an airfoil to the speed of the airfoil through the fluid, the density of the fluid and the circulation around the airfoil. The Kutta–Joukowski theorem is a fundamental theorem in aerodynamics used for the calculation of lift of an airfoil and any two-dimensional bodies including circular cylinders translating in a uniform fluid at a constant speed large enough so that the flow seen in the body-fixed frame is steady and unseparated.

JSTOR ( May 2015) ( Learn how and when to remove this template message).

Unsourced material may be challenged and removed.įind sources: "Kutta–Joukowski theorem" – news Please help improve this article by adding citations to reliable sources. This vortex formed is called as starting vortex.This article needs additional citations for verification. To maintain conservation of forces, circulation of the equal and opposite strength sets and leaves from the trailing edge of the airfoil (Kelvin's circulation theorem). Which hints the presence of circulation around an airfoil which displaces the stagnation point. This condition is known as the Kutta condition. It was observed that stagnation point near the trailing edge which is on the upper surface of the airfoil initially, always moves exactly at the trailing edge (Shown in figure b). As the angle of attack of an airfoil is increased stagnation point moves toward the lower surface of the airfoil. One near the leading edge of the airfoil and other near the trailing edge on the upper surface of the airfoil (As shown in figure a). In a case of an airfoil, at the very initial stage of the flow two stagnation points set in (Stagnation point is the highest static pressure location on the airfoil where flow separates or rejoins). This velocity difference causes static pressure difference above and below the cylinder which results in a lift in a rotating cylinder.

JOUKOWSKI AIRFOIL FREE

On the other hand, the lower part of the cylinder which moves toward the left slows down the free velocity. The upper part of the cylinder which moves toward right boosts the free stream velocity just above it in the same direction. Clockwise circulation sets around the cylinder due to its rotation. It is easy to visualize lift produced due to circulation around the rotating cylinder.

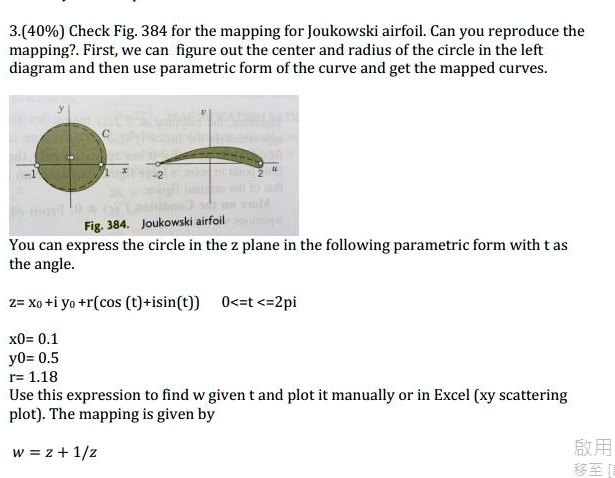
Let's begin with the rotating cylinder in the uniform flow field. This boundary layer is instrumental in the wing’s ability to produce lift. These layers of air where the effect of viscosity is significant near the airfoil surface altogether are called a 'Boundary Layer'. This happens till air velocity reaches almost the same as free stream velocity. Similarly, the air layer with reduced velocity tries to slow down the air layer above it and so on. Due to the viscous effect, this zero-velocity fluid layer slows down the layer of the air just above it. The air close to the surface of the airfoil has zero relative velocity due to surface friction (due to Van der Waals forces). Zoom closely into what is happening on the surface of the wing. The rightmost term in the equation represents circulation mathematically and is evaluated using vector integrals. Understand lift production, let us visualize an airfoil (cut section of a Mathematical Formulation of Kutta-Joukowski Theorem: The theorem relates the lift produced by a two-dimensional object to the velocity of the flow field, the density of flow field, and circulation on the contours of the wing.